The Perpetual Race of Piranesi and the Tortoise
Melting reality, ancient history and fantasy into one, the etchings of Giovanni Battista Piranesi hold an unparalleled allure that continues to entrance and captivate. They offer an escape into imagined and unknown worlds; each drawing an organism of its own, containing an immense depth of spatial layering and an extraordinary sense of scale that ventures unerringly towards the sublime. Particularly intense in this respect is the plan for Piranesi’s project Collegio Romano.
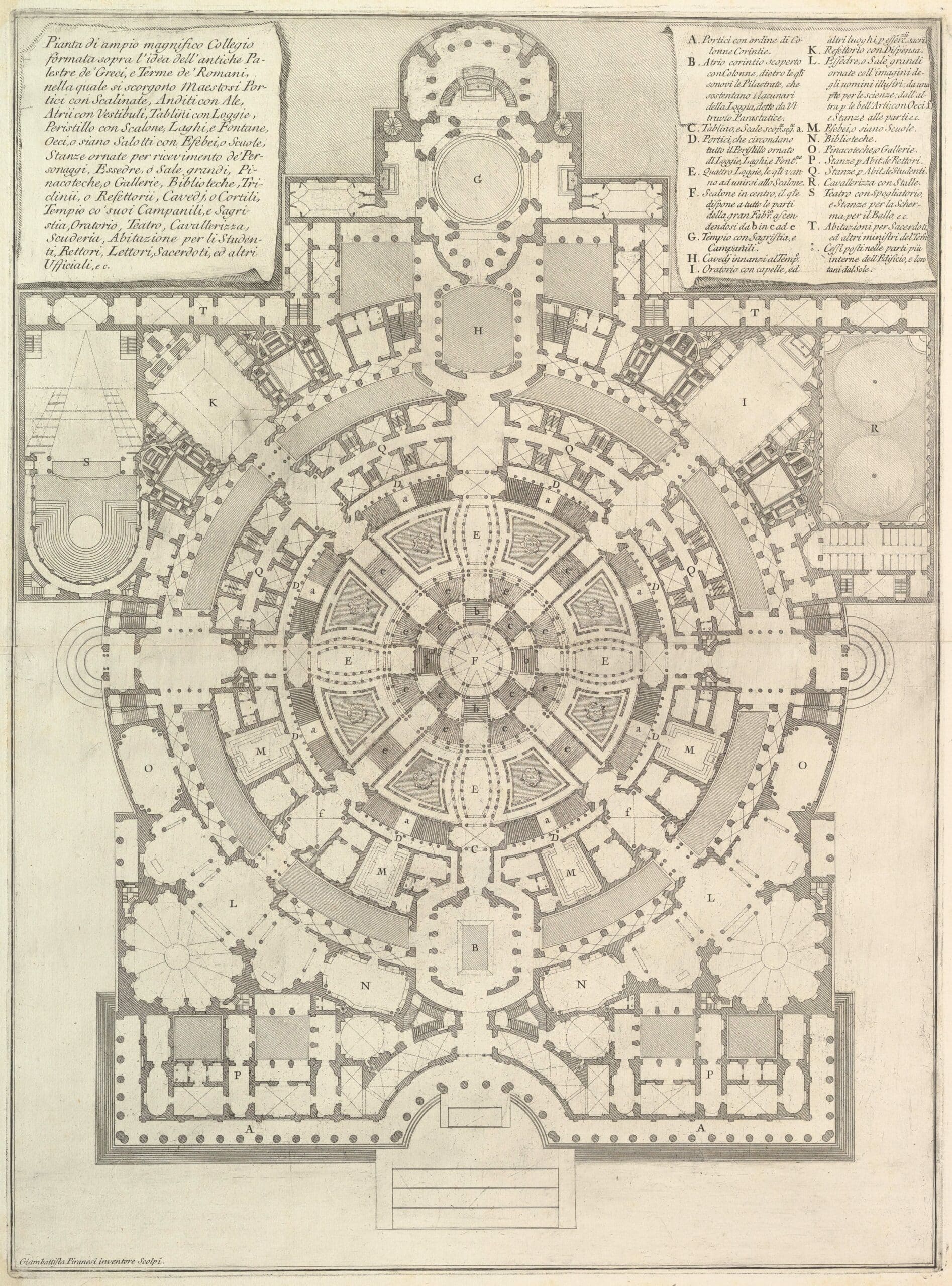
The circular, centralised plan describes a complex spatial arrangement with a grand, almost scaleless, civic quality that matches the full title of the work, Pianta di ampio magnifico Collegio (Plan of a Spacious and Magnificent College). The plan is certainly vast and magnificent yet its functionality is far less evident than its grandeur. The title, Collegio, therefore seems like a disguise for a mysterious unknown. The plan’s enigma is heightened by the fact that it is the only known articulation of this project. It was, however, published alongside a drawing of a grand port, Parte di ampio magnifico Porto (below) in Opere Varie di Archiettura, in which a majestic circular, colosseum-like building appears at once to be both descending from the heavens and rising from the billowing smoke of the city. If not a direct representation of the Collegio, its scale and curved, concentric form certainly echo the plan.

Though Piranesi’s plan is formally reminiscent of ancient Greek gymnasia and Roman Baths, it fills the viewer with great intrigue but no real understanding of the project. As one attempts to navigate this dense labyrinth of spaces and stairs, any sense of conventional or comprehensible functionality seems to evaporate. One is lost in a flower-like explosion of pure geometry where symmetry and centrality dominate entirely. The focus of the viewer is concentrated on the centre of the plan with such an intensity that the drawing appears like a portal to another realm. Like a precise piece of machinery, the concentric rings of the plan could begin to rotate at any moment; the teeth of these interlocking cogs clicking into place as they spin faster and faster, the burning core eventually opening to reveal a world beyond. Even as a completely static 250-year-old etching incapable of teleportation, Collegio Romano remains deeply intriguing and offers a point of entry into the enigmatic imagination of Piranesi.
If this plan cannot be readily understood as a project for a working building, one might consider it as an exploration of the infinite. The dominant symbolism of the circle is so stark that this reading may seem almost too obvious, yet its use here is not straightforward. The circle is employed as a device to concentrically arrange a dense matrix of spaces around a central, jewel-like chamber. The circle’s purity is not, however, celebrated at the centre point as might be expected. One does not find a grand void or cupola here but instead, a curious eight-pointed star form labelled Scalone (staircase), the dashed lines of which suggest walkways or stairs bridging above to meet in the middle. The centre of Piranesi’s plan, to which all the energy in this drawing is pulled, is not a destination. Arrival to this point leads only to seven new ways back into this maze of a plan. The only prize Piranesi offers here is an invitation to return to the vast concentric network of passages, colonnades, stairs and atria conjured by his imagination.
The rotunda in this instance doesn’t speak of transcendence and perfection in form, as it does in the Pantheon, Bramante’s Tempietto or Schinkel’s Altes Museum. Collegio Romano’s centre point is, rather, a portal to further, seemingly limitless, exploration and spatial encounter. Piranesi’s cryptic project is an unbounded and speculative subdivision of space imagined and communicated through the infinite possibility offered by the act of drawing. Considered in this way, Collegio Romano, embodies the presence of the infinite that pervades Piranesi’s entire oeuvre. From his Carceri to Campo Marzio, his works are boundless, both spatially and with regards to time. History and reality are not sacred and serve only as starting points for Piranesi’s infinite imagination.
The notion of infinity with regards to architecture is as enticing and liberating to some as it is problematic and worrisome for others. Space is by its very essence infinite and our fundamental task as architects is to subdivide it in meaningful ways. We speak about it in casual paradoxes, as both an entity and non-entity, something we can shape and mould, but never grasp, something we can divide yet never truly measure. In order to concisely consider Piranesi’s relationship with infinity, and the role of drawing within this, perhaps another paradox might help.
The Greek philosopher Zeno of Elea (c. 490–430 BC) describes an epic, unending race between Achilles, the symbol of speed, and the tortoise, the symbol of slowness. The tortoise is given a head-start of ten metres because Achilles runs ten times faster. In the time it takes Achilles to cover those first ten metres the tortoise travels one metre. Achilles runs this next metre but the tortoise is now ten centimetres further ahead. Achilles runs these ten centimetres and the tortoise runs one. Continued ad infinitum Achilles’ pursuit of the tortoise will never end. The discussion and refutation of Zeno’s paradox have prevailed and, rather than debate this further, we might instead simply place our original hero, Piranesi, in the feather-weight, Alphafly shoes of Achilles.
Piranesi was certainly as prolific and mercurial in the production of etchings as Achilles was fleet-footed, but perhaps Piranesi wasn’t concerned with catching the tortoise. To do so would mean reaching a defined endpoint, achieving perfection. His work does not, however, demonstrate such preoccupations. Constantly evolving and striving toward the unknown, it could be read as a pursuit of the infinite; something unreachable but a consistent source of sustenance for his passion and imagination.
Throughout ancient mythology the tortoise has endured as a symbol of tranquility and stability. A giant tortoise is depicted in Hindu culture with four elephants standing on its shell, who are in turn carrying the Earth on their backs. Piranesi, seemingly aware of their significance also incorporated tortoises into his work, here, supporting the base of his lavish design for three marble candelabras. More importantly, though, it seems that in the acceptance of indeterminacy in his work he found an equally stable grounding for his practice.

Piranesi’s etchings demonstrate serene contentment in the infinite possibility offered by the act of drawing in the exploration of space. Collegio Romano offers an optic into this extraordinary spatial practice. The plan has an immensely powerful presence yet it remains an elusive and ethereal suggestion of a building. Its infinitesimal indeterminacy lends an unquantifiable and slightly decadent sense of mystique to the plan. It is opulent in its eschewal of function; Collegio Romano is an assemblage of visual signs that are known to represent elements of architecture but are here simply arranged like jewels in a crown. They have been placed with precision and care to form a constellation that is kaleidoscopic in its beauty but is no more than a symbol of architecture. Piranesi’s Collegio Romano forms a cosmos in which ancient architecture finds itself swirling in an infinite continuum with architecture that is still standing and architecture that can never be.
This investigation of Piranesi’s Collegio Romano should not be understood as a call to arms for utopian paper projects, but simply a reminder of the interrogative aspect of drawing and the infinitesimal possibility it affords. Admittedly, this prospect can be daunting and intimidating so it is perhaps helpful to reflect on the words of a writer who was deeply comfortable with the subject of infinity. Jorge Luis Borges, to whom the title and content of this essay are deeply indebted, wrote ‘The precise quantity of points in the universe is the same as in a metre in the universe, or in a decimetre, or in the deepest trajectory of a star.’
Piranesi’s Collegio Romano seems to represent an attempt to capture the latter yet even this seemingly infinite subdivision of space occurs within the confines of a sheet slightly smaller than DIN-A1. Just as Borges describes the eternal presence of infinity in space, the size of the page and the thickness of the line cannot limit the extent of the imagination and the power of the idea. Seeking to capture something that is constantly edging out of reach ensures a constant sense of becoming remains present in the design and drawing process. Without this, these acts become formulaic and predictable. Drawing and designing ought to be approached with less expectancy in a defined outcome and rather a greater acceptance of an indeterminate one. To do so grants the freedom to explore ideas we don’t yet fully understand; ideas that shift, grow and are reborn as we draw them. The completed drawing, in this case, serves only as the catalyst for further thought. A chance for the tortoise to creep further ahead.
Originally from Belfast, Marc currently lives and works in Berlin. He is interested in the infinite worlds we collect through the act of drawing.
This text was entered into the 2020 Drawing Matter Writing Prize. Click here to read the winning texts and more writing that was particularly enjoyed by the prize judges.
